Analysis of anvil centering accuracy of cubic press based on small displacement torsor theory
-
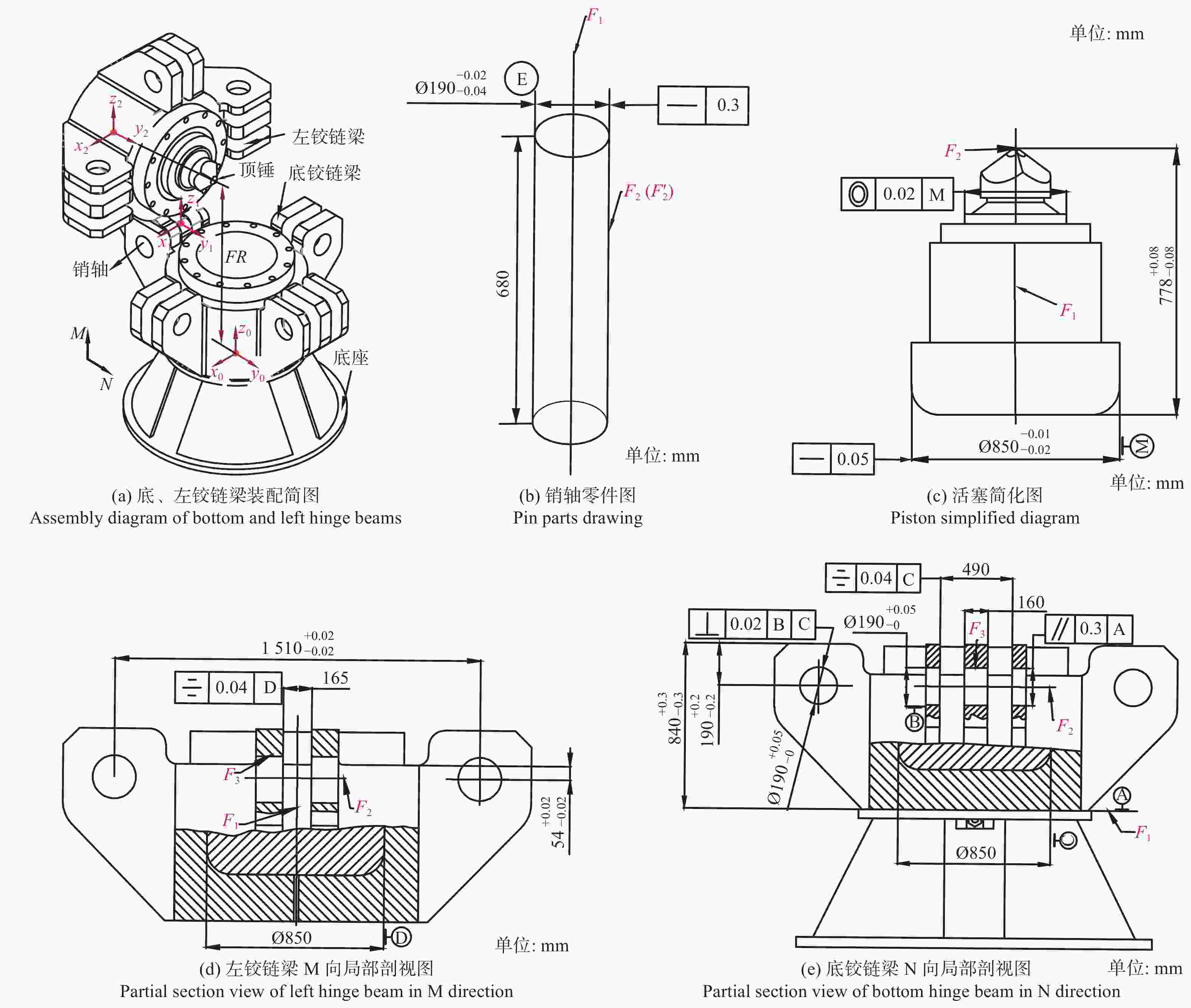
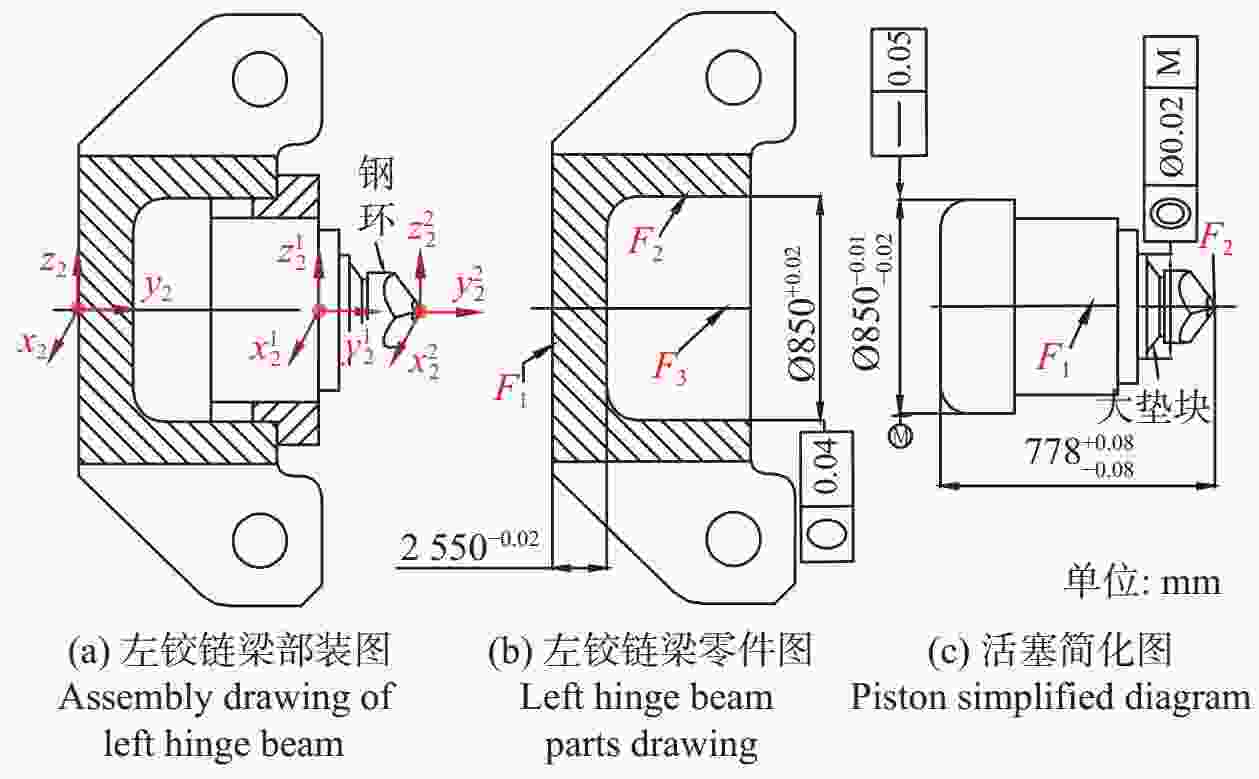
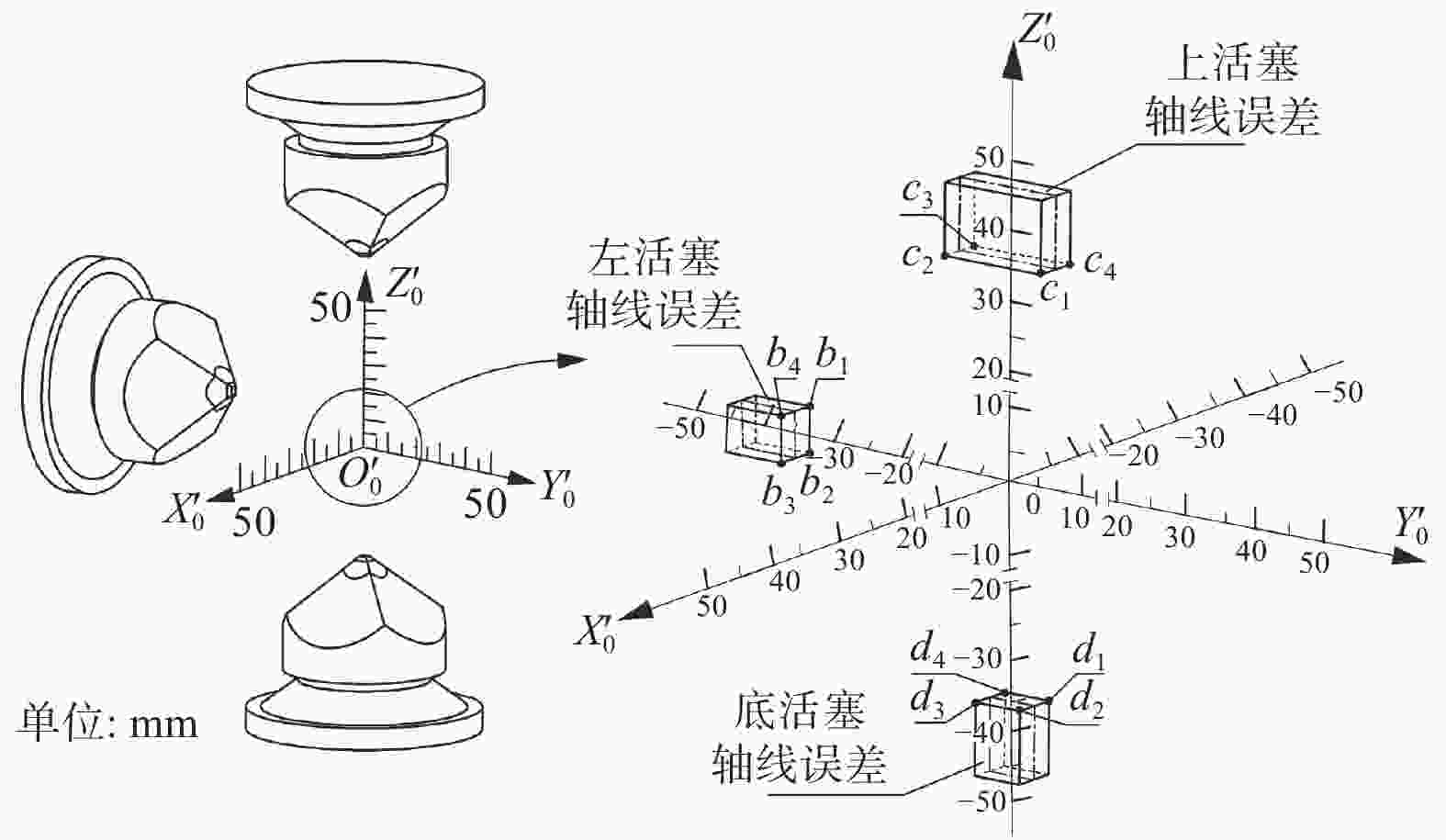
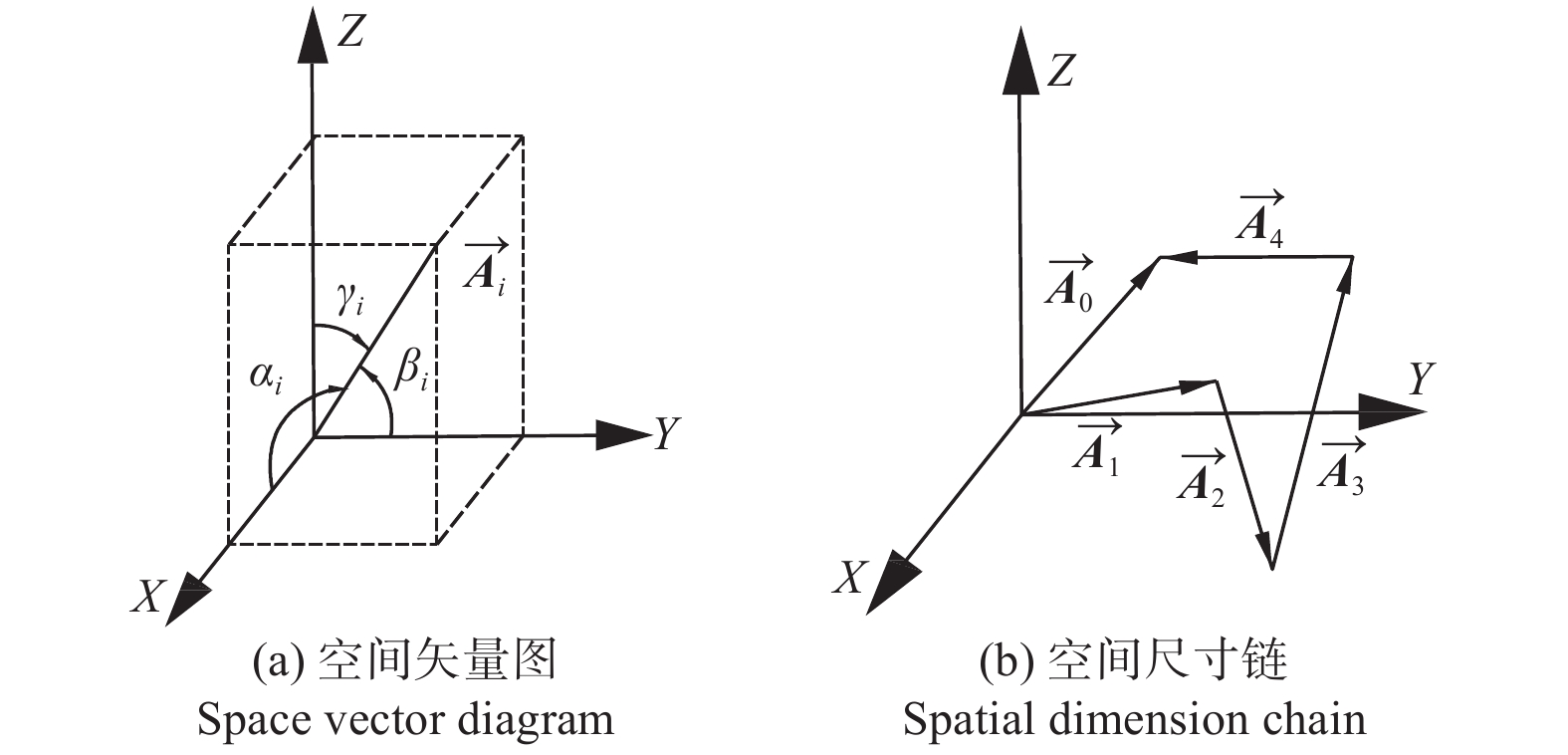
摘要: 为了提升金刚石合成装备六面顶压机顶锤的对中精度,开展六面顶压机铰链梁的工作腔体装配误差分析。首先,基于小位移旋量(small displacement torsor,SDT)理论,建立要素采用不同公差原则时的金刚石压机铰链梁装配公差模型;其次,利用空间矢量表示三维尺寸链,基于空间矢量环叠加原理推导出表示铰链梁活塞顶锤运动位姿的封闭环尺寸及其变动计算模型,进而得到其底、左、上顶锤中心轴线与各自顶锤外端面交点可能的误差范围;最后,比较三维公差分析得到的单个铰链梁活塞顶锤位姿累积闭环误差FR与一维尺寸链分析得到的类似误差X。结果表明:由于FR的组成环要多于X的组成环,其结果更能准确地表示铰链梁系统的误差传递结果,且FR[−1.005, 1.005]表示的误差范围要大于X[−1.000, 0.780]表示的误差范围,验证了该方法对装配公差分析的优越性和准确性。同时,通过筛选试验设计(Plackett-Burman design,PBD)筛选出对单个铰链梁活塞顶锤位姿封闭环影响较显著的变量,为合理分配压机铰链梁的加工精度提供了理论基础,有利于保障金刚石压机顶锤的对中精度,合理分配压机铰链梁相关结构配合公差及各部件的容差,并优化设备的加工成本。Abstract: Objectives: The diamond synthetic equipment in China is mainly the hinged cubic press (referred to as cubic press). With the acceleration of large-scale presses, the performance of cubic presses has greatly improved, but higher requirements have also been put forward for the assembly accuracy of these presses. In order to improve the centering accuracy of the top hammer of the cubic press, the assembly errors of the working cavity of the hinge beam for the cubic press are researched. Methods: Firstly, based on the small displacement torsor (SDT) theory, the assembly tolerance model of the hinge beam of the cubic press with different tolerance principles is established. Secondly, the space vector is used to represent the three-dimensional dimension chain. Based on the space vector ring superposition principle, the closed ring size and its variation calculation model representing the motion posture of the hinge beam piston top hammer is derived, and the possible error range of the intersection points between the bottom, the left, and the upper top hammer axes and their respective top hammer outer end faces are obtained. Finally, the cumulative closed-loop error FR obtained from the three-dimensional tolerance analysis of the single hinge beam piston top hammer posture is compared with the similar error X1 obtained from the one-dimensional dimensional chain analysis. At the same time, the Plackett-Burman design (PBD) is used to screen out the variables that have a significant effect on the sealing ring of the top hammer posture of a single hinge beam piston. Results: (1) Through the calculation of the three-dimensional tolerance analysis method established by the cubic press, it is found that the possible errors of the axis of the top hammer of the left hinge beam are [−0.070, 0.095] in the X direction, [−0.655, 0.655] in the Y direction, and [−0.855, 1.035] in the Z direction. The possible errors of the axis of the top hammer of the bottom hinge beam are [−0.030, 0.055] in the X and Y directions, and [0.080, 0.100] in the Z direction. The possible errors of the axis of the top hammer of the upper hinge beam are [−0.111, 0.135] in the X direction, [−1.180, 1.155] in the Y direction, and [−1.820, 1.915] in the Z direction. (2) The dimensional variation error X1 of the hydraulic cylinder axis of the left hinge beam in the Z direction is compared and calculated by using the one-dimensional dimensional chain. The variation error X1 of the closed ring is [−1.000, 0.780] when the dimensional chain extreme value method is used for analysis, and the variation error X1 of the closed ring is [−0.930, 0.410] when the Monte Carlo method is used for analysis. The calculated result of the Monte Carlo method is less than that of the extreme value method. This is because the calculation assumes that the tolerances of each part follow a normal distribution, which is more in line with the actual production situation and closer to the actual assembly error. (3) When the diameter of the pin adopts the principle of independence, the possible position error of the size of the hydraulic cylinder axis of the left hinge beam in the Z direction is [−1.005, 1.005]. When the diameter of the pin is marked by the inclusion principle, the position error changes to [−0.855, 0.980]. From the comparison of results, the use of different tolerance principles leads to different tolerance analysis results. (4) The Plackett-Burman design (PBD) is used to screen out four highly significant variables, namely, the parallelism tolerance corresponding to variable M1, the dimensional tolerance corresponding to variable M2, and the straightness tolerance corresponding to variables M5 and M7, which have a great impact on the precision of the hinge beam. Conclusions: Based on SDT theory, the three-dimensional tolerance analysis method under different tolerance principles is established for the cubic press, and the possible error variation ranges of the bottom, left and upper hinge beam top hammer axes are calculated respectively. By comparing the errors obtained by the three-dimensional analysis method with those obtained by the one-dimensional dimensional chain method, it is found that the former has a larger error range than the latter, which proves that the three-dimensional analysis model method used in this paper is superior to the one-dimensional tolerance analysis method. At the same time, when the pin diameter is marked with different tolerance principles, the axis error of the hinge beam hydraulic cylinder is calculated, and the error range corresponding to the inclusion principle is smaller than that corresponding to the independent principle. That is to say, when the inclusion principle is applied in the pin diameter marking, the position variation error of the hinge beam hydraulic cylinder axis can be ensured to be smaller, which is more in line with the high-precision requirements of the diamond cubic press. Finally, the four highly significant variables that have great influence on the precision of the hinge beam are selected, providing a theoretical basis for the reasonable distribution of the machining precision of the press hinge beam.
-
表 1 典型几何公差带的SDT模型及其矢量约束[11]
Table 1. SDT model and its vector description for typical geometric tolerance zones[11]
公差带 公差带形状 SDT模型 约束 圆柱面 
$ \left[ {\begin{array}{*{20}{c}} u&v&0 \\ {\Delta \alpha }&{\Delta \beta }&0 \end{array}} \right] $ $ \begin{gathered} u = \left[ { - \frac{t}{2},\frac{t}{2}} \right],v = \left[ { - \frac{t}{2},\frac{t}{2}} \right],\Delta \alpha = \left[ { - \frac{t}{L},\frac{t}{L}} \right] \\ \Delta \beta = \left[ { - \frac{t}{L},\frac{t}{L}} \right] \\ {\left( {u + \frac{{\Delta \alpha \cdot L}}{2}} \right)^2} + {\left( {v + \frac{{\Delta \beta \cdot L}}{2}} \right)^2} = \left[ {0,{{\left( {\frac{t}{2}} \right)}^2}} \right] \\ \end{gathered} $ 两平行平面 
$\left[ {\begin{array}{*{20}{c}} 0&{\text{0}}&\omega \\ {\Delta \alpha }&{\Delta \beta }&0 \end{array}} \right]$ $ \begin{gathered} \omega = \left[ { - \frac{t}{2},\frac{t}{2}} \right],\Delta \alpha = \left[ { - \frac{t}{2},\frac{t}{2}} \right],\Delta \beta = \left[ { - \frac{t}{{{L_2}}},\frac{t}{{{L_2}}}} \right] \\ \left| \omega \right| + \left| {\frac{{\Delta \alpha \cdot {L_1}}}{2}} \right| + \left| {\frac{{\Delta \beta \cdot {L_2}}}{2}} \right| = \left[ {0,\frac{t}{2}} \right] \\ \end{gathered} $ 表 2 组成环的SDT模型及其约束
Table 2. SDT models and its constraints for constituting rings
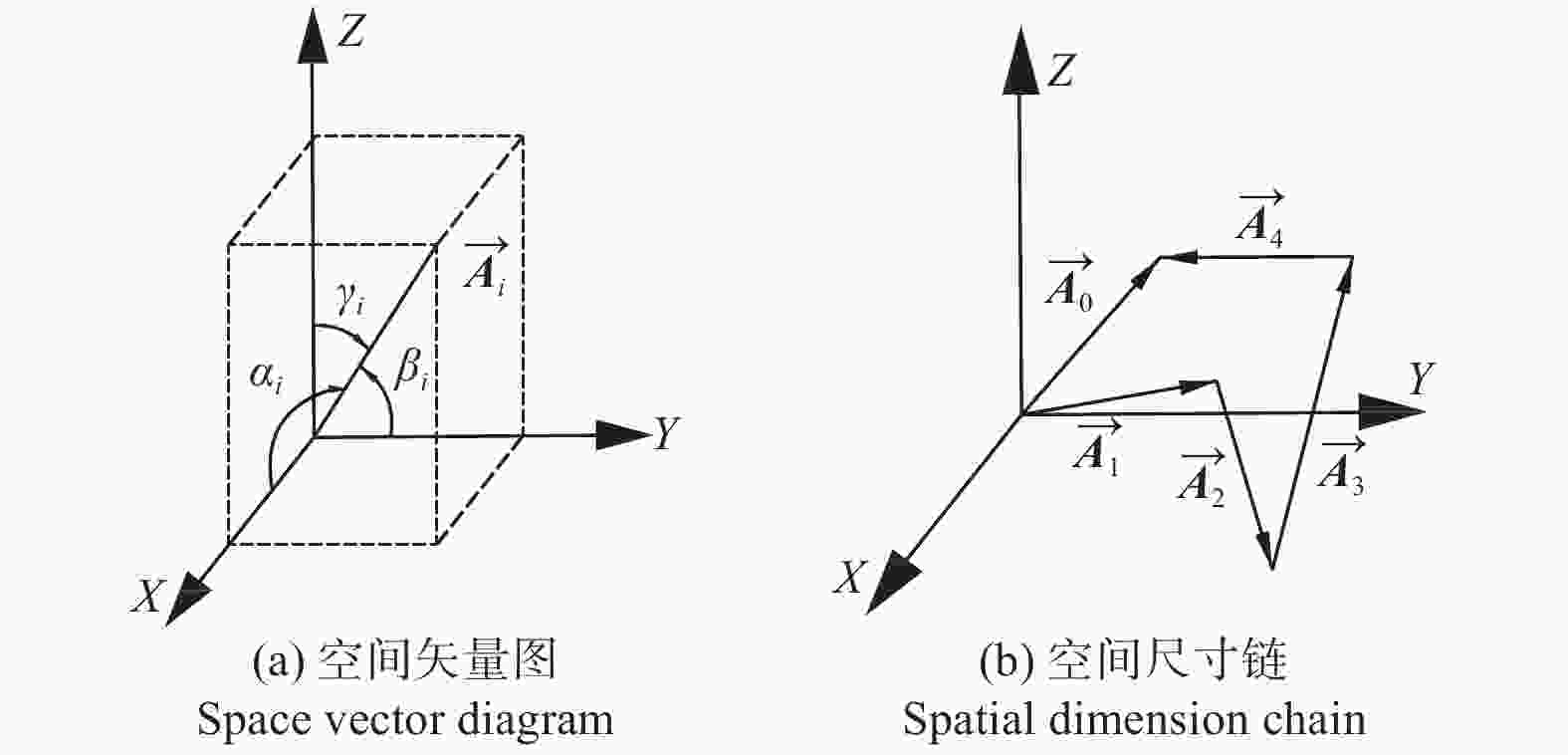
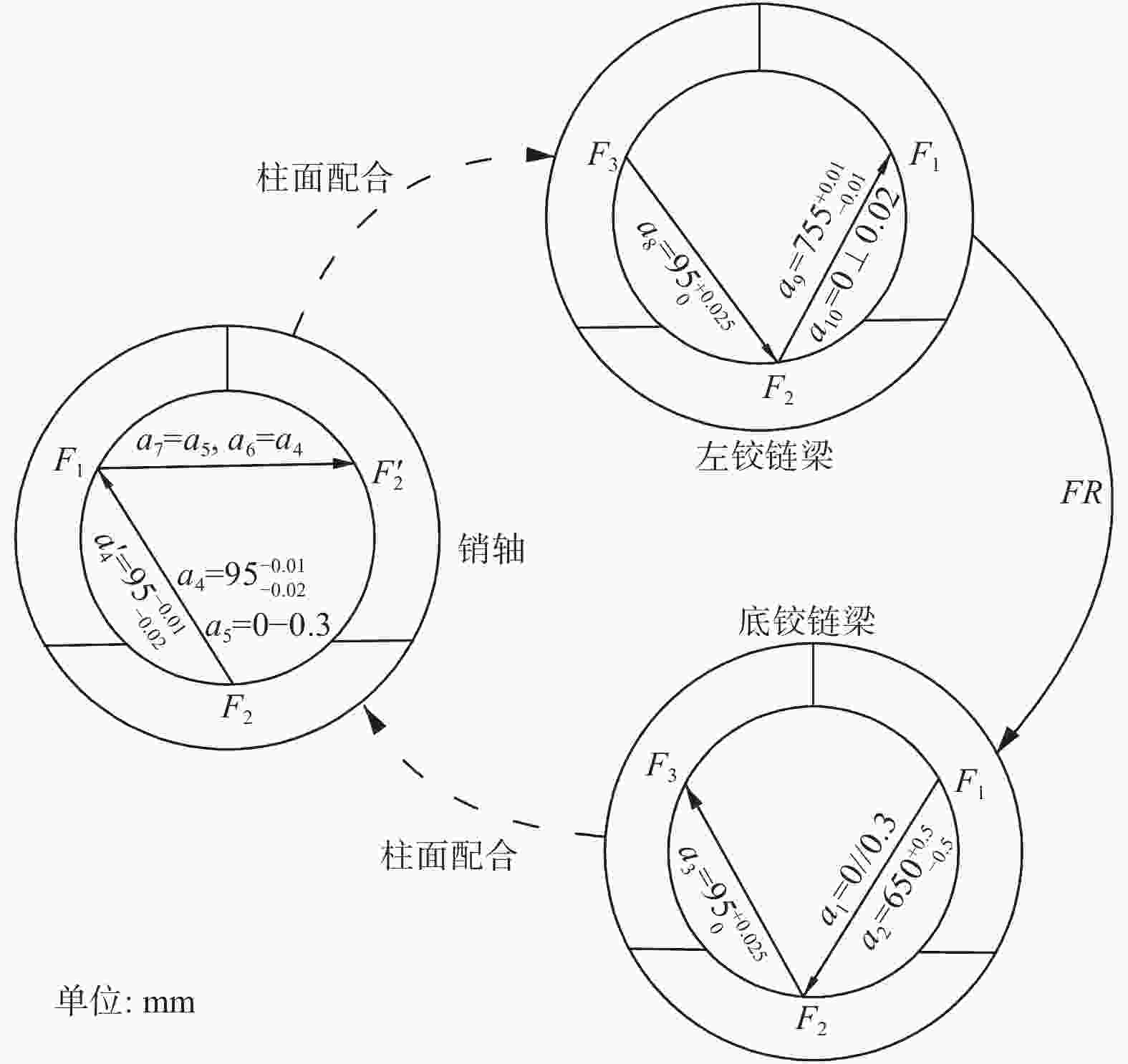
各环尺寸及其$ {\alpha }_{{i}}、{\beta }_{{i}}、{\gamma }_{i} $ SDT模型 约束 $\begin{gathered} {a_1} = 0 \\ {\alpha _1} = {90^\circ},{\beta _1} = {90^\circ},{\gamma _1} = {0^\circ} \\ \end{gathered} $ $\left[ {\begin{array}{*{20}{c}} 0&0&\omega \\ {\Delta \alpha }&{\Delta \beta }&0 \end{array}} \right]$ $\begin{gathered} \omega = \left[ { - \frac{{0.3}}{2},\frac{{0.3}}{2}} \right] \\ \Delta \alpha = \Delta \beta = \left[ { - \frac{{0.3}}{{680}},\frac{{0.3}}{{680}}} \right] \\ \end{gathered} $ $\begin{gathered} {a_2} = 650_{ - 0.5}^{ + 0.5} \\ {\alpha _2} = {90^\circ},{\beta _2} = {90^\circ},{\gamma _2} = {0^\circ} \\ \end{gathered} $ $\left[ {\begin{array}{*{20}{c}} 0&0&\omega \\ 0&0&0 \end{array}} \right]$ $\omega = \left[ { - 0.5,0.5} \right]$ $ \begin{gathered} {a_3} = 95_0^{0.025} \\ {\alpha _3} = {90^\circ},{\beta _3} = {90^\circ},{\gamma _3} = {0^\circ} \\ \end{gathered} $ $\left[ {\begin{array}{*{20}{c}} 0&0&\omega \\ 0&0&0 \end{array}} \right]$ $\omega = \left[ {0,0.025} \right]$ $ \begin{gathered} {a_4} = 95_{ - 0.02}^{ - 0.01} \\ {\alpha _4} = {90^\circ},{\beta _4} = {90^\circ},{\gamma _4} = {180^\circ} \\ \end{gathered} $ $\left[ {\begin{array}{*{20}{c}} 0&0&\omega \\ 0&0&0 \end{array}} \right]$ $\omega = \left[ { - 0.02, - 0.01} \right]$ $ \begin{gathered} a_{_4}' = 95_{ - 0.02}^{ - 0.01} \\ \alpha _{_4}' = {90^\circ},\beta _{_4}' = {90^\circ},\gamma _{_4}' = {180^\circ} \\ \end{gathered} $ $\left[ {\begin{array}{*{20}{c}} 0&0&\omega \\ 0&0&0 \end{array}} \right]$ $\omega = \left[ { - 0.02, - 0.01} \right]$ $\begin{gathered} {a_5} = 0 \\ {\alpha _5} = {90^\circ},{\beta _5} = {90^\circ},{\gamma _5} = {180^\circ} \\ \end{gathered} $ $\left[ {\begin{array}{*{20}{c}} 0&v&\omega \\ 0&{\Delta \beta }&{\Delta \gamma } \end{array}} \right]$ $\begin{gathered} v = \omega = \left[ { - \frac{{0.3}}{2},\frac{{0.3}}{2}} \right] \\ \Delta \beta = \Delta \gamma = \left[ { - \frac{{0.3}}{{680}},\frac{{0.3}}{{680}}} \right] \\ \end{gathered} $ $ \begin{gathered} {a_6} = 95_{ - 0.02}^{ - 0.01} \\ {\alpha _6} = {90^\circ},{\beta _6} = {90^\circ},{\gamma _6} = {0^\circ} \\ \end{gathered} $ $\left[ {\begin{array}{*{20}{c}} 0&0&\omega \\ 0&0&0 \end{array}} \right]$ $\omega = \left[ { - 0.02, - 0.01} \right]$ $\begin{gathered} {a_7} = 0 \\ {\alpha _7} = {90^\circ},{\beta _7} = {90^\circ},{\gamma _7} = {0^\circ} \\ \end{gathered} $ $\left[ {\begin{array}{*{20}{c}} 0&v&\omega \\ 0&{\Delta \beta }&{\Delta \gamma } \end{array}} \right]$ $\begin{gathered} v = \omega = \left[ { - \frac{{0.3}}{2},\frac{{0.3}}{2}} \right] \\ \Delta \beta = \Delta \gamma = \left[ { - \frac{{0.3}}{{680}},\frac{{0.3}}{{680}}} \right] \\ \end{gathered} $ $ \begin{gathered} {a_8} = 95_0^{ + 0.025} \\ {\alpha _8} = {90^\circ},{\beta _8} = {90^\circ},{\gamma _8} = {180^\circ} \\ \end{gathered} $ $\left[ {\begin{array}{*{20}{c}} 0&0&\omega \\ 0&0&0 \end{array}} \right]$ $\omega = \left[ {0,0.025} \right]$ $ \begin{gathered} {a_9} = 755_{ - 0.01}^{ + 0.01} \\ {\alpha _9} = {90^\circ},{\beta _9} = {90^\circ},{\gamma _9} = 0^\circ \\ \end{gathered} $ $\left[ {\begin{array}{*{20}{c}} 0&0&\omega \\ 0&0&0 \end{array}} \right]$ $\omega = \left[ { - 0.01,0.01} \right]$ $\begin{gathered} {a_{10}} = 0 \\ {\alpha _{10}} = {90^\circ},{\beta _{10}} = {90^\circ},{\gamma _{10}} = {0^\circ} \\ \end{gathered} $ $\left[ {\begin{array}{*{20}{c}} 0&v&\omega \\ 0&{\Delta \beta }&{\Delta \gamma } \end{array}} \right]$ $\begin{gathered} v = \omega = \left[ { - \frac{{0.02}}{2},\frac{{0.02}}{2}} \right] \\ \Delta \beta = \Delta \gamma = \left[ { - \frac{{0.3}}{{755}},\frac{{0.3}}{{755}}} \right] \\ \end{gathered} $ 表 3 2种公差分析结果对比
Table 3. Comparison of two tolerance analysis results
维数 方法 取值 / mm 一维 极值法 [−1.000,0.780] 蒙特卡罗法 [−0.930,0.410] 三维 独立原则 [−1.005,1.005] 包容原则 [−0.855,0.980] 表 4 PBD试验变量水平表和编码
Table 4. PBD test variable level table and coding
变量 编码 低水平 / mm 高水平 / mm N1 A −0.300 0.300 N2 B −0.500 0.500 N3 C 0 0.025 N4 D −0.020 −0.010 N5 E −0.300 0.300 N6 F −0.020 −0.010 N7 G −0.300 0.300 N8 H 0 0.025 N9 J −0.010 0.010 N10 K −0.020 0.020 表 5 PBD试验组合及响应
Table 5. PBD test combination and response
试验号 A B C D E F G H J K 封闭环
FRi / mm1 0.300 0.500 0 −0.010 0.300 −0.020 0.300 0.025 0.010 −0.020 0.135 2 0.300 0.500 0 −0.010 0.300 −0.010 −0.300 0 −0.010 0.020 0.350 3 0.300 −0.500 0 −0.020 −0.300 −0.020 −0.300 0 −0.010 −0.020 −0.670 4 0.300 0.500 0.025 −0.020 −0.300 −0.020 0.300 0 0.010 0.020 0.995 5 0.300 0.500 0.025 −0.020 0.300 −0.010 0.300 0 −0.010 −0.020 0.365 6 0.300 0.500 0 −0.020 −0.300 −0.010 −0.300 0.025 0.010 −0.020 0.635 7 0.300 −0.500 0.025 −0.010 0.300 −0.020 −0.300 0 0.010 −0.020 −0.635 8 0.300 −0.500 0 −0.010 −0.300 −0.010 0.300 0 0.010 0.020 −0.330 9 0.300 −0.500 0 −0.020 0.300 −0.020 0.300 0.025 −0.010 0.020 −0.375 10 0.300 −0.500 0.025 −0.020 0.300 −0.010 −0.300 0.025 0.010 0.020 −0.920 11 0.300 −0.500 0.025 −0.010 −0.300 −0.010 0.300 0.025 −0.010 −0.020 −0.070 12 0.300 0.500 0.025 −0.010 −0.300 −0.020 −0.300 0.025 −0.010 0.020 0.340 表 6 回归分析结果
Table 6. Regression analysis results
来源 模型 A B C E G H J K 平方和 3.8200 0.2700 3.0000 0.0018 0.2700 0.2700 0.0018 0.0012 0.0012 自由度 8 1 1 1 1 1 1 1 1 均方根 0.480000 0.270000 3.000000 0.001800 0.270000 0.270000 0.001875 0.001200 0.001200 F值 2 385.09 1 350.00 1 5000.00 9.38 1 350.00 1 350.00 9.38 6.00 6.00 p值 < 0.0001 < 0.0001 < 0.0001 0.0549 < 0.0001 < 0.0001 0.0549 0.0917 0.0917 显著性 高度显著 高度显著 高度显著 高度显著 高度显著 -
[1] 何文斌, 姚港生, 汪曙光, 等. 基于响应面法的缸梁一体式六面顶压机铰链梁优化 [J]. 金刚石与磨料磨具工程, 2023, 43(4): 523-530. doi: 10.13394/j.cnki.jgszz.2022.0204HE Wenbin, YAO Gangsheng, WANG Shuguang, et al. Optimization of the link of the cylinder-beam integrated six-face top pressure machine using response surface methodology [J]. Diamond & Abrasives Engineering, 2023, 43(4): 523-530. doi: 10.13394/j.cnki.jgszz.2022.0204 [2] 张永亮, 黄琪元, 王书楠, 等. 基于雅可比旋量的风电机组驱动链装配误差分析 [J]. 太阳能学报,2022,43(12):242-247. doi: 10.19912/j.0254-0096.tynxb.2021-0740ZHANG Yongliang, HUANG Qiyuan, WANG Shunan, et al. Assembly error analysis of wind turbine drive chain based on Jacobi spino [J]. Acta Energiae Solaris Sinica,2022,43(12):242-247. doi: 10.19912/j.0254-0096.tynxb.2021-0740 [3] CHEN H, LI X, JIN S. A statistical method of distinguishing and quantifying tolerances in assemblies [J]. Computers & Industrial Engineering,2021,156:107259. doi: 10.1016/j.cie.2021.107259 [4] MUJEZINOVIC A, DAVIDSON J K, SHAH J J. A new mathematical model for geometric tolerances as applied to polygonal faces [J]. Journal of Mechanical Design,2004,126(3):504-518. doi: 10.1115/1.1701881 [5] CLEMENT A, RIVIERE A. Tolerancing versus nominal modeling in next generation CAD/CAM system: Proceedings of 3rd CIRP seminar on computer aided tolerancing [C]. CIRP: 1993. [6] TURNER J U, WOZNY M J. The M-space theory of tolerances: Proceedings of the ASME 16th design automation conference [C]. ASME: 1990. [7] 任彦松, 马志赛, 王晓鹏, 等. 含间隙飞行器折叠舵面的尺寸链分析 [J]. 航空科学技术,2023,34(3):89-96. doi: 10.19452/j.issn1007-5453.2023.03.013REN Yansong, MA Zhisai, WANG Xiaopeng, et al. Dimensional chain analysis of folded rudder surface of aircraft with clearance [J]. Aerospace Science and Technology,2023,34(3):89-96. doi: 10.19452/j.issn1007-5453.2023.03.013 [8] FAN J, WANG P, ZHANG H, et al. Research on tolerance modeling method and 3D tolerance analysis technology based on STD: IOP conference series: Materials science and engineering [C]. Sanya: IOP, 2019. [9] 熊峰, 李刚炎, 王平俊, 等. 基于雅可比旋量的并联配合特征三维公差分析方法及其应用 [J]. 组合机床与自动化加工技术,2019(11):13-16, 21. doi: CNKI:SUN:ZHJC.0.2019-11-004XIONG Feng, LI Gangyan, WANG Pingjun, et al. 3D tolerance analysis method of parallel fit feature based on Jacobi spinor and its application [J]. Modular Machine Tool & Automatic Manufacturing Technique,2019(11):13-16, 21. doi: CNKI:SUN:ZHJC.0.2019-11-004 [10] DENIS T, VINCENT D. Taking into account form variations in polyhedral approach in tolerancing analysis [J]. Procedia CIRP,2018(5):75-81. doi: 10.1016/j.procir.2018.04.084 [11] 穆晓凯, 孙清超, 孙克鹏, 等. 基于载荷作用的柔性体三维公差建模及精度影响分析 [J]. 机械工程学报,2018,54(11):39-48. doi: 10.3901/JME.2018.11.039MU Xiaokai, SUN Qingchao, SUN Kepeng, et al. Three-dimensional tolerance modeling and accuracy impact analysis of flexible bodies based on load action [J]. Chinese Journal of Mechanical Engineering,2018,54(11):39-48. doi: 10.3901/JME.2018.11.039 [12] 周志鹏, 彭和平, 常素萍. 考虑公差原则应用的统计公差分析方法 [J]. 机械设计与制造, 2022(12): 38-43. doi: 10.19356/j.cnki.1001-3997.2022.12.014ZHOU Zhipeng, PENG Heping, CHANG Suping. The statistical tolerance analysis method taking the application of tolerance principle into account [J]. Machinery Design & Manufacture, 2022(12): 38-43. doi: 10.19356/j.cnki.1001-3997.2022.12.014 [13] 孟巧凤, 张林鍹, 尹琳峥, 等. 基于3DCS的三维尺寸公差的分析与优化 [J]. 系统仿真学报,2018,30(5):1730-1738. doi: 10.16182/j.issn1004731x.joss.201805014MENG Qiaofeng, ZHANG Linxuan, YIN Linzheng, et al. Analysis and optimization of 3D dimensional tolerances based on 3DCS [J]. Journal of system simulation,2018,30(5):1730-1738. doi: 10.16182/j.issn1004731x.joss.201805014 [14] BOURDET P, CLEMENT A. A study of optimal-criteria identification based on the small displacement screw model [J]. Annals of the CIRP,1988,37(1):503-506. doi: 10.1016/S0007-8506(07)61687-4 [15] BOURDET P, MATHIEU L, LARTIGUE C, et al. The concept of the small displacement torsor in metrology [J]. Advanced Mathematical Tool in Metrology, Advances in Mathematics for Applied Sciences,1996,40:110-122. [16] 方东阳, 李秀明, 张琳娜, 等. 基于GPS的形位公差项目分析和设计 [J]. 郑州大学学报(工学版),2005,3(3):73-75. doi: 10.3969/j.issn.1671-6833.2005.03.019FANG Dongyang, LI Xiuming, ZHANG Linna, et al. Analysis and design of form and position tolerance items based on GPS [J]. Journal of Zhengzhou University(Engineering Edition),2005,3(3):73-75. doi: 10.3969/j.issn.1671-6833.2005.03.019 [17] HÉNON M. The Monte Carlo method [J]. Astrophysics and Space Science, 1971, 14(1): 151-167. -



 下载:
下载:



 邮件订阅
邮件订阅 RSS
RSS
