塔形组合式金刚石圆盘锯能耗建模及参数优化

郭安顺; 张进生; 张恒; 王凯达; 孙钰虎; 牛平平; 王一彩

doi:10.13394/j.cnki.jgszz.2023.0128

塔形组合式金刚石圆盘锯能耗建模及参数优化

doi: 10.13394/j.cnki.jgszz.2023.0128

郭安顺^{1, 2, 3},
张进生^{1, 2, 3, 4, ,},
张恒^{1, 2, 3, 4},
王凯达^{1, 2, 3},
孙钰虎^{1, 2, 3},
牛平平⁴,
王一彩⁴

1.
山东大学机械工程学院, 济南 250061
2.
山东省石材工程技术研究中心, 济南 250061
3.
高效洁净机械制造教育部重点实验室(山东大学), 济南 250061
4.
山东大学日照研究院, 山东日照 276800

基金项目: 山东省科技型中小企业创新能力提升工程项目（2022TSGC2179）；山东省自然科学基金资助项目（ZR2023QE162）；日照市重点研发计划项目（2021ZDYF010109）；日照市自然科学基金项目（RZ2021ZR35）。

详细信息

通讯作者:
张进生，男，1962年生，教授、博士研究生导师。主要研究方向：石材制品高效绿色加工技术与装备。E-mail：zhangjs@sdu.edu.cn

中图分类号: TG580；TG74
计量
- 文章访问数: 484
- HTML全文浏览量: 204
- PDF下载量: 17
- 被引次数: 0
出版历程
- 收稿日期: 2023-06-12
- 修回日期: 2023-08-07
- 录用日期: 2023-09-04
- 网络出版日期: 2023-11-06
- 刊出日期: 2024-06-28

Energy consumption modeling and parameter optimization of tower combined diamond circular saw blade

GUO Anshun^{1, 2, 3},
ZHANG Jinsheng^{1, 2, 3, 4
, ,},
ZHANG Heng^{1, 2, 3, 4},
WANG Kaida^{1, 2, 3},
SUN Yuhu^{1, 2, 3},
NIU Pingping⁴,
WANG Yicai⁴

1.
School of Mechanical Engineering, Shandong University, Jinan 250061, China
2.
Stone Engineering Research Center of Shandong Province, Jinan 250061, China
3.
Education Key Laboratory of Efficient Clean Machinery Ministry (Shandong University), Jinan 250061, China
4.
Rizhao Intelligent Manufacturing Institute of Shandong University, Rizhao 276800, Shandong, China

摘要

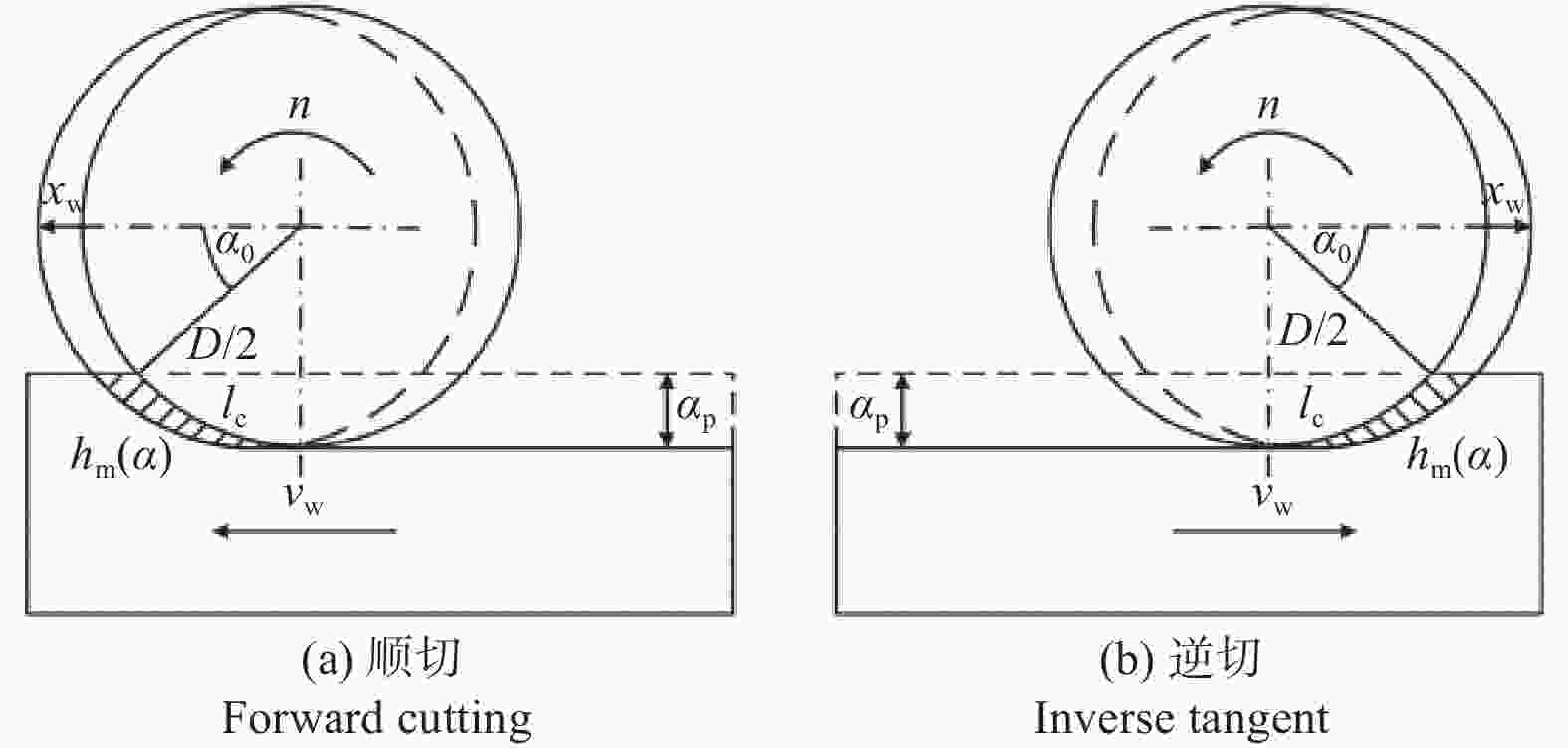
摘要: 为准确预测塔形组合式金刚石圆盘锯在荒料锯切过程中的功率，以组合锯切系统中单锯片的单磨粒平均未变形切屑厚度为媒介，建立了锯切功率的参数模型，并对其进行修正，提出一种少样本快速预测模型，通过锯切实验测量不同参数组合下的锯切功率，采用多元线性回归方法拟合数据以获取可靠的模型系数。最后以锯切参数为优化变量，以锯切比能和锯切时间最小为优化目标建立优化模型，并采用改进粒子群算法对模型进行优化求解。试验结果表明，参数模型充分解释了各锯切参数对锯切功率的影响，能够准确预测不同锯片组合方式下的锯切功率，改进的粒子群算法有较高的优化性能，使用优化后的参数能够显著降低锯切功率。
- 塔形组合式金刚石圆盘锯 /
- 能耗模型 /
- 平均未变形切屑厚度 /
- 参数优化
Abstract: To precisely forecast the power of a tower combined diamond circular saw blade during material sawing, a model for sawing power was established using the average thickness of an undeformed chip from a single grinding grain of an individual saw blade within the combination saw as a medium. This model was then refined to enhance accuracy. A fast and accurate prediction model, requiring only a small number of samples, was developed. Sawing power was measured through various parameter combinations via sawing experiments, and model coefficients were obtained by fitting the data using multivariate linear regression techniques. An optimization model was subsequently established, with sawing parameters as optimization variables. The objectives of this model were to minimize sawing specific energy and reduce sawing time. An optimized particle swarm algorithm was adopted to solve the model. The experimental results reveal that the parameter model can comprehensively elucidate the influence of various sawing parameters on sawing power, with the model accurately forecasting the sawing power under different saw blade combinations. The improved particle swarm algorithm demonstrates strong optimization performance, with optimized parameters contributing to significant reductions in sawing power.
- tower combined diamond circular saw /
- energy consumption model /
- average undeformed chip thickness /
- parameter optimization

HTML全文

图 1 组合锯示意图

Figure 1. Diagram of combination saw

下载: 全尺寸图片幻灯片

图 2 单颗磨粒未变形切屑厚度变化示意图

Figure 2. Schematic diagram of the variation in undeformed chip thickness of a single abrasive particle

下载: 全尺寸图片幻灯片

图 3 功率随锯切参数变化示意图

Figure 3. Schematic diagram of power variation with sawing parameters

下载: 全尺寸图片幻灯片

图 4 锯切功率与h_m的关系示意图

Figure 4. Diagram of relationship between sawing power and h_m

下载: 全尺寸图片幻灯片

图 5 3P3W接线图

Figure 5. 3P3W wiring diagram

下载: 全尺寸图片幻灯片

图 6 不同直径锯片组合锯切方式

Figure 6. Combined sawing mode with different diameters

下载: 全尺寸图片幻灯片

图 7 改进粒子群算法求解流程

Figure 7. The solving process of particle swarm optimization algorithm.

下载: 全尺寸图片幻灯片

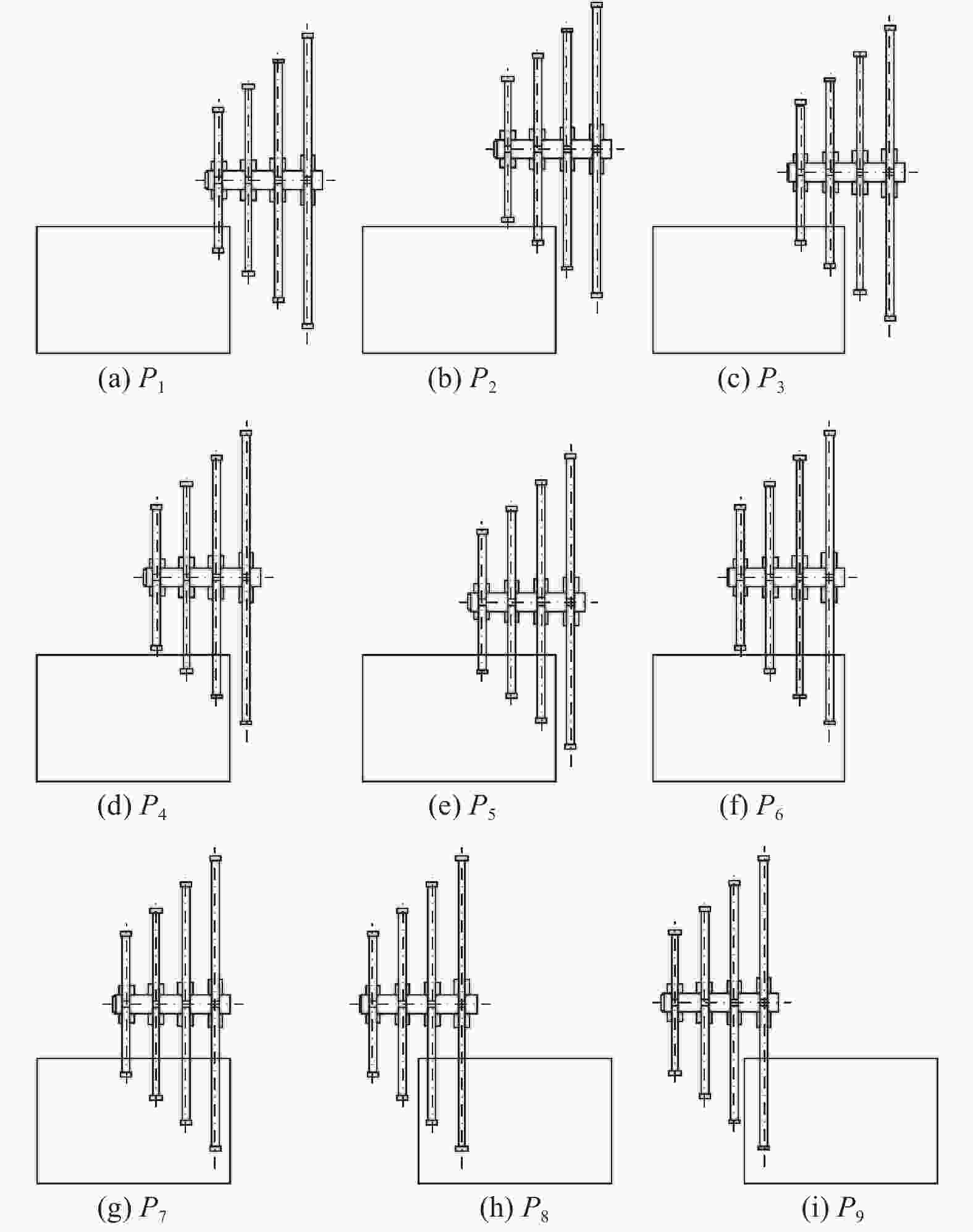
表 1 锯切参数组合

Table 1. Sawing parameter combination

参数	取值
转速 n / (r·min⁻¹)	380，395，410
进给速度 v_w / (m·min⁻¹)	3.45，4.45，5.45
锯切深度 a_p / mm	4，6，8

下载: 导出CSV

表 2 锯切能耗测量结果

Table 2. Measurement results of sawing energy consumption

编号	转速 n / (r·min⁻¹)	进给速度 v_w / (m·min⁻¹)	锯切深度 a_p / mm	后3片组合锯切功率 P₆ / kW	4片组合锯切功率 P₇ / kW
1	380	3.45	4	12.74	15.20
2	380	4.45	4	15.66	17.33
3	380	5.45	4	18.89	21.56
4	380	3.45	6	16.22	19.11
5	380	4.45	6	19.50	22.92
6	380	5.45	6	22.59	27.04
7	380	3.45	8	19.72	22.74
8	380	4.45	8	22.97	26.97
9	380	5.45	8	26.81	31.96
10	395	3.45	4	13.29	15.57
11	395	4.45	4	15.74	18.84
12	395	5.45	4	18.90	22.87
13	395	3.45	6	16.93	19.58
14	395	4.45	6	19.69	23.32
15	395	5.45	6	23.44	27.52
16	395	3.45	8	19.59	22.80
17	395	4.45	8	23.31	27.73
18	395	5.45	8	27.84	32.62
19	410	3.45	4	13.51	16.58
20	410	4.45	4	15.96	19.29
21	410	5.45	4	18.91	22.42
22	410	3.45	6	16.84	19.79
23	410	4.45	6	19.78	23.99
24	410	5.45	6	23.47	27.94
25	410	3.45	8	19.83	23.32
26	410	4.45	8	23.59	28.35
27	410	5.45	8	28.38	35.44

下载: 导出CSV

表 3 P₆模型拟合系数

Table 3. Fitting coefficients of the P₆model

系数	Beta1	Beta2	Sig	VIF
k_cp6	0.144		<0.001
K₆	0.248		0.033
x	0.543		<0.001	1.000
a	0.078	0.055	<0.001	1.000
b	0.204	0.655	<0.001	1.000
c	−0.210	0.750	<0.001	1.000
R²	99.4%		<0.001
DW	2.355
RMSE	0.017

下载: 导出CSV

表 4 P₇模型拟合系数

Table 4. Fitting coefficients of the P₇ model

系数	Beta1	Beta2	Sig	VIF
k_cp7	0.171		<0.001
K₇	0.027		<0.001
x	0.543		<0.001	1.000
a	−0.312	0.109	<0.001	1.000
b	0.228	0.660	<0.001	1.000
c	−0.205	0.737	<0.001	1.000
R²	99.0%		<0.001
DW	1.808
RMSE	0.022

下载: 导出CSV

表 5 P₆模型对比分析

Table 5. Comparative analysis of the P₆ model

编号	转速 n / (r·min⁻¹)	进给速度v_w / (m·min⁻¹)	锯切深度a_p / mm	P_预测 / kW	P_实测 / kW	η / %
1	370	3.45	8	18.97	18.40	3.10
2	385	4.45	6	19.80	20.97	5.58
3	400	5.45	4	18.61	19.29	3.53

下载: 导出CSV

表 6 P₇模型对比分析

Table 6. Comparative analysis of the P₇ model

编号	转速 n / (r·min⁻¹)	进给速度v_w / (m·min⁻¹)	锯切深度a_p / mm	P_预测 / kW	P_实测 / kW	η / %
1	370	3.45	8	21.53	23.25	7.40
2	385	4.45	6	22.94	24.03	4.54
3	400	5.45	4	21.95	22.95	4.35

下载: 导出CSV

表 7 优化结果对比分析

Table 7. Comparative analysis of optimization results

编号	方案	转速 n / (r·min⁻¹)	进给速度 v_w / (m·min⁻¹)	锯切深度 a_p / mm	F
1	LPSO	378.78	6.22	8.22	0.043
1	NPSO	371.82	5.49	8.96	0.038
2	LPSO	375.24	5.91	8.54	0.039
2	NPSO	370.30	6.18	8.27	0.037
3	LPSO	378.16	5.69	8.69	0.043
3	NPSO	372.39	5.38	9.07	0.038

下载: 导出CSV

表 8 优化解与经验解对比分析

Table 8. Comparative analysis of optimal solution and empirical solution

方法	转速 n / (r·min⁻¹)	进给速度v_w / (m·min⁻¹)	锯切深度 a_p / mm	锯切比能 SEC / (kJ·cm⁻³)	加工时间 T / min
优化	370.30	6.18	8.27	0.695	9.783
经验	410.00	5.45	8.00	0.757	11.468

下载: 导出CSV

参考文献(15)

[1]	TURCHETTA S. Cutting force and diamond tool wear in stone machining [J]. The International Journal of Advanced Manufacturing Technology,2012,61(5):441-448.
[2]	POLINI W, TURCHETTA S. Force and specific energy in stone cutting by diamond mill [J]. International Journal of Machine Tools and Manufacture,2004,44(11):1189-1196. doi: 10.1016/j.ijmachtools.2004.04.001
[3]	黄国钦, 徐西鹏. 基于锯切弧区切向力分布的功率消耗模型 [J]. 机械工程学报,2011,47(21):170-176. doi: 10.3901/JME.2011.21.170 HUANG Guoqin, XU Xipeng. Establishment of power model for circular sawing based on tangential force distribution at the contact zone [J]. Chinese Journal of Mechanical Engineering,2011,47(21):170-176. doi: 10.3901/JME.2011.21.170
[4]	HUANG G, ZHANG M, HUANG H, et al. Estimation of power consumption in the circular sawing of stone based on tangential force distribution [J]. Rock Mechanics and Rock Engineering,2018,51(4):1249-1261. doi: 10.1007/s00603-017-1380-2
[5]	张昆, 田业冰, 丛建臣, 等. 基于动态惯性权重粒子群算法的磨削低能耗加工方法 [J]. 金刚石与磨料磨具工程,2021,41(1):71-75. ZHANG Kun, TIAN Yebing, CONG Jianchen, et al. Reduce grinding energy consumption by modified particle swarm optimization based on dynamic inertia weigh [J]. Diamond & Abrasives Engineering,2021,41(1):71-75.
[6]	陈行政, 李聪波, 吴磊, 等. 面向能耗的多刀具孔加工刀具直径及工艺参数集成优化模型 [J]. 机械工程学报,2018,54(15):221-231. doi: 10.3901/JME.2018.15.221 CHEN Xingzheng, LI Congbo, WU Lei, et al. Integrating optimization of cutter diameter and cutting parameters for energy-aware multi-tool hole machining [J]. Chinese Journal of Mechanical Engineering,2018,54(15):221-231. doi: 10.3901/JME.2018.15.221
[7]	黄国钦, 黄辉, 郭桦, 等. 串珠绳锯切花岗石过程中锯切参数对锯切力和能耗的影响 [J]. 机械工程学报,2009,45(3):234-239. doi: 10.3901/JME.2009.03.234 HUANG Guoqin, HUANG Hui, GUO Hua, et al. Influences of sawing parameters on forces and energy in wire sawing of granite [J]. Journal of Mechanical Engineering,2009,45(3):234-239. doi: 10.3901/JME.2009.03.234
[8]	KONSTANTY J. Theoretical analysis of stone sawing with diamonds [J]. Journal of Materials Processing Technology,2002,123(1):146-154. doi: 10.1016/S0924-0136(02)00071-7
[9]	LI K, LIAO W. Modelling of ceramic grinding processes part I. Number of cutting points and grinding forces per grit [J]. Journal of Materials Processing Technology,1997,65(1/2/3):1-10.
[10]	ZHOU J, WANG K, ZHANG J, et al. Rock breakage and tools performance during rock processing by multidiameter combination saw with different diameters [J]. Rock Mechanics and Rock Engineering,2022,55(7):4459-4476. doi: 10.1007/s00603-022-02824-9
[11]	周京国. 塔形组合式金刚石圆锯片锯切特性研究[D]. 济南: 山东大学, 2022. ZHOU Jingguo. Sawing performance of tower combined diamond circular saw blade[D]. Jinan: Shandong University, 2022.
[12]	帅茂杭, 熊国江, 胡晓, 等. 基于改进多目标骨干粒子群算法的电力系统环境经济调度 [J]. 控制与决策,2022,37(4):997-1004. SHUAI Maohang, XIONG Guojiang, HU Xiao, et al. Economic emission dispatch of power system based on improved bare-bone multi-objective particle swarm optimization algorithm [J]. Control and Decision Making,2022,37(4):997-1004.
[13]	陈贵敏, 贾建援, 韩琪. 粒子群优化算法的惯性权值递减策略研究 [J]. 西安交通大学学报,2006(1):53-56,61. CHEN Guimin, JIA Jianyuan, HAN Qi. Study on the strategy of decreasing inertia weight in particle swarm optimization algorithm [J]. Journal of Xi'an Jiaotong University,2006(1):53-56,61.
[14]	贾会群, 魏仲慧, 何昕, 等. 基于改进粒子群算法的路径规划 [J]. 农业机械学报,2018,49(12):371-377. JIA Huiqun, WEI Zhonghui, HE Xin, et al. Path Planning Based on Improved Particle Swarm Optimization Algorithm [J]. Journal of Agricultural Machinery,2018,49(12):371-377.
[15]	毛开富, 包广清, 徐驰. 基于非对称学习因子调节的粒子群优化算法 [J]. 计算机工程,2010,36(19):182-184. MAO Kaifu, BAO Guangqing, XU Chi. Particle swarm optimization algorithmbasedon non-symmetric learning factor adjusting [J]. Computer Engineering,2010,36(19):182-184.